An alternative derivation of the relativistic mass formula can be made using Newton‘s Second Law of Motion in connection with the mass-energy equivalence principle E=mc².
Heuristic explanation of the dependence of mass on velocity
Before proving the dependence of mass on velocity, we want to give a coherent explanation for it.
Experiments in particle accelerators show a phenomenon that seems inexplicable by Newtonian mechanics:
The inertia of particles increases with speed.
In this article we will see that this phenomenon is a consequence of the principle of equivalence (E=mc²) of mass and energy. The latter can be derived from the laws of classical physics (see here).
By deriving the mass-energy equivalence, we have shown that the absorption of radiant energy by a physical body is accompanied by an increase in the mass of the body itself.
Assuming that E is the energy absorbed, the increase in mass is equal to the quotient E / c².
Considering the law of energy conservation, one can extend this property to all other forms of energy as follows:
An energy absorption causes a mass increase of a physical system according to the mass-energy equivalence E = mc².
The latter also happens when an unbound body is subjected to an external force.
In this case, acceleration takes place, with a concomitant increase in kinetic energy and hence mass.
In quantitative terms, this concept results in the following identity:
Mass of the moving body = mass of the body at rest + mass of the kinetic energy of the body.
Consequentially:
The inertia of a moving body depends on its kinetic energy
An increase in velocity hence causes an increase in the mass of the “system” made up of the body and its energy.
The dependence of mass on velocity is therefore a direct consequence of the principle of equivalence between mass and energy.
With this concept we deviate on this website from the established proofs of the relativistic mass formula.
The interpretation of Lorentz and Einstein is based on the length contraction and is therefore, in my opinion, difficult to understand.
Instead, an increase in mass due to an increase in kinetic energy is easily plausible.
This also implies a direct connection between Newtonian and relativistic mechanics.
Having said that, we can now proceed with the derivation of the relativistic mass formula.
After the derivation of E=mc² using classical physics, this is the second proof of fundamental importance for the purposes of this website.
In fact, with the following derivation of the relativistic mass formula, we obtain the first relation containing the Lorentz factor.
We therefore enter the field of application of the Theory of Relativity starting from Newtonian mechanics, without assuming the postulate of the constancy of the speed of light and without using the Lorentz transformations.
On the other hand, the relativistic mass formula is the fundamental relation in the alternative path that we are discussing here.
In fact, we use the relativistic mass formula to derive all the other proofs, including the theoretical one of the constancy of the speed of light.
Description of the proof in a reduced form
(For the detailed version of the proof, see the fifth chapter of the book “Newton and Relativity“).
If the displacement ds runs in the same direction as the force F acting on a body, then from the relation of the Second Law of Motion1 …
\[ \vec{F} = \frac{d(m\vec{v})}{dt} = m\frac{d\vec{v}}{dt} + \vec{v}\frac{dm}{dt} \]… we can directly derive the following differential equation for work and energy:
\[ Fds = dE = mvdv + v^2dm\]Note that the term v²dm allows the hypothesis of a variable mass, as actually occurs at high speeds.
If we replace the added kinetic energy dE with the equivalent mass term c²dm and integrate the resulting differential equation, …
\[c^2dm = mvdv + v^2dm\] \[\frac{dm}{m} = \frac{v}{c^2-v^2}dv\] \[\int_{m_{0}}^m\frac{dm}{m} = \int_0^v\frac{v}{c^2-v^2}dv\] \[[ln(m)]_{m_{0}}^m=-\frac{1}{2}[ln(c^2-v^2)]_0^v\] \[ln\frac{m}{m_0}=\frac{1}{2}ln\frac{c^2}{c^2-v^2}\] \[\frac{m}{m_0}=\sqrt{\frac{c^2}{c^2-v^2}}\]… we obtain the relativistic mass formula:
\[ m = \frac{m_{0}}{\sqrt{1-\frac{v^{2}}{c^{2}}}} \]where:
\[ \frac{1}{\sqrt{1-\frac{v^{2}}{c^{2}}}} \]is the Lorentz factor.
This shows the compatibility of Newton’s Second Law of Motion with Relativity.
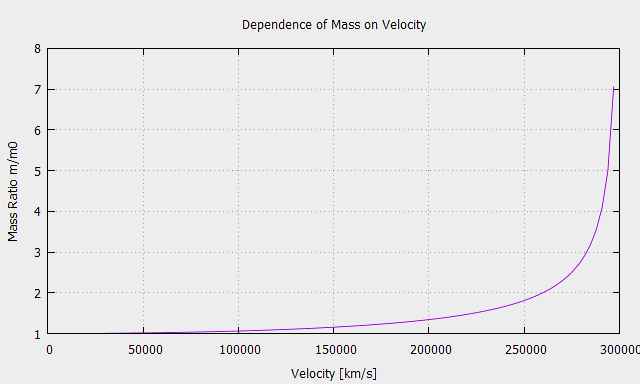
The consequent use of variable mass in Newton’s law shows that the Special Theory of Relativity can be seen as a logical extension of Newtonian mechanics.
The application of the principles of conservation of energy and momentum using relativistic mass enables the alternative derivation of the relationships of Special Relativity (in this regard, see the page: “Sequence of Relativistic Proofs“).
You find the detailed version of this alternative derivation of the relativistic mass formula in the fifth chapter of the book “Newton and Relativity“.
With the Relativistic Calculator you can calculate the Lorentz factor as a function of speed.
Continue on the alternative path of relativistic proofs: Alternative derivation of relativistic energy.
______________
The aim of this website is to introduce the theory of relativity using a new, simple and accessible demonstration method.
To support this project, please order the book “Newton and Relativity“.
______________
Footnotes:
- Newton’s Second Law of Motion is used consistently in its original form, according to which the force is equal to the time derivative of the momentum. The product rule gives the sum of two terms. At low speeds the mass can be considered constant, so the second term of the sum can be set equal to zero. At high speeds, however, this term must be calculated on the basis of the dependence of mass on velocity. ↩︎



Thank You ! I was thinking there must be a way to explain it without mathematics..by feeling it..this article enabled me to feel it !
I was pleased to receive your comment.
If I have succeeded in explaining the relativistic dependence of mass on velocity in a more accessible way, then I have achieved my goal with this article.